Worm Gear Design Calculation Pdf

logobossplant.netlify.com › ★ Worm Gear Design Calculation Pdf Files ★

To calculate a worm gear with center distance 100 mm. The worm has 2 teeth, and the worm wheel has 41 teeth. The axial/transverse module is 4. The pressure angle at the normal section is 20°. The worm's facewidth is 60 mm. You should select a sensible facewidth for the worm wheel. The axis tolerance is js7.

ZAR3 Worm Gear Calculation ZAR3+ Software for Cylindrical Worm Gear Design (C) Copyright 1993-2017 by HEXAGON Software, Berlin Bases for Calculation ZAR3 calculates all dimensions of cylindrical worm gear pairs with ZI, ZA, ZK, ZN or ZH worms, as well as efficiency, tooth forces and the safety margins against root fatigue fracture and pitting. Pre-dimensioning In pre-dimensioning recommendations are made for axial distance, modulus and number of teeth based on gear ratio, drive power, drive rotational speed and material. The recommendations can be used in the following geometry and strength calculations. Geometry Calculation In dimensioning you can adjust the recommended values to match available sizes or company standards. Or by varying the axial distance ratio and addendum modification you can determine the optimum worm gear for your application.

Strength Calculation The strength calculation computes the factors SF (fatigue fracture) and SH (pitting) along with tooth forces on the worm and worm gear, as well as the efficiency of the gear. Efficiency ZAR3 calculates the toothing efficiency, toothing and idling loss of power. The program provides recommended values and help graphics for determination of the tooth friction value. Material Data Base The program already includes a data base containing the most important gear materials and their values.

Users of the ZAR1+ spur gear and ZAR2 bevel gear programs can access this as a common data base. Tooth Forces Axial and radial forces, as well as tangential force and normal force are calculated. These values can be transfered to the WL1/WL1+ software for shaft calculation. Plus-Version ZAR3+ The extended version ZAR3+ provides additional functions: ZAR3+ generates true-scale drawing of worm and worm gear.

ZAR3+ provides an additional input window for modifications of tooth height factors and profile shift coefficient x. These functions are useful for design of complementary gears of steel worm and plastic worm gear. ZAR3+ calculates tooth thickness and over pin/ball diameters (OPD). You can enter pin diameter and flank tolerances or select from tolerance system according to DIN 3967.

CAD Interface A true-scale drawing of worm and worm wheel, as well as tables with the gear data can be exported to CAD via the DXF or IGES interfaces. The drawings can of course also be displayed on screen and printed out. Import/Export Text printout can be generated as HTML table and exported to Excel. Input data may be loaded from an Excel worksheet.

The mating worm gear teeth have a helical lead. (Note: The name “worm wheel” is often used interchangeably with “worm gear”.) A central section of the mesh, taken through the worm's axis and perpendicular to the worm gear's axis, as shown in Figure 9-2, reveals a rack-type tooth of the worm, and a curved involute tooth form for the worm. . Worm gears are used when larggge gear reductions are needed. It is common for worm gears to have reductions of 20:1, and even up to 300:1 or greater. Many worm gears have an interesting property that no other gear set has: the worm can easily turn the gear but the gear cannot turn the wormgear, but the gear cannot turn the worm. Set the Design Guide to Module, Center Distance to 13.5 mm and Pressure Angle to 22.5 degrees. Set the gear option to Component and enter 12 for the Number of Teeth of Gear 1 and 42 for the Number of Teeth of Gear 2. Also set the Facewidth to 5 mm and Unit Correction to 0.1. Click Calculation to initiate the necessary calculations to. Gear Design National Broach and Machine Division,of Lear Siegler, Inc. A gear can be defined as a toothed wheel which, when meshed with another toothed wheel with similar configura-tion, will transmit rotation from one shaft to another. Depending upon the type and accuracy of motion desired, the gears and the profiles of the gear teeth can be.

HEXAGON Help System For all entries a help text or auxiliary picture can be displayed. For example, there are diagrams for input of the tooth friction value µz0 in relation to lubricant and average slide speed. There are also diagrams for optimizing the diameter-to-center distance ratio dm1/a in relation to fatigue stress or efficiency. Users can modify and append help texts and auxiliary pictures. When error messages occur you can have an error description and remedy suggestion displayed.

Hardware and Software Requirements ZAR3+ is available as 32 bit and 64 bit application for Windows 7, Windows 8, Windows 10. Scope of Delivery CD-ROM or zip file for download with program and pdf manual, License agreement for indefinite period of time. Information and Update Service HEXAGON Software is continuously improved and updated. Licensed users can obtain new versions at the update price.

Worm Gear Design Calculation Pdf Files Pdf

Each program is provided with an individual license number and user files. No extra charge is made to registered users for telephonic support and hotline use.

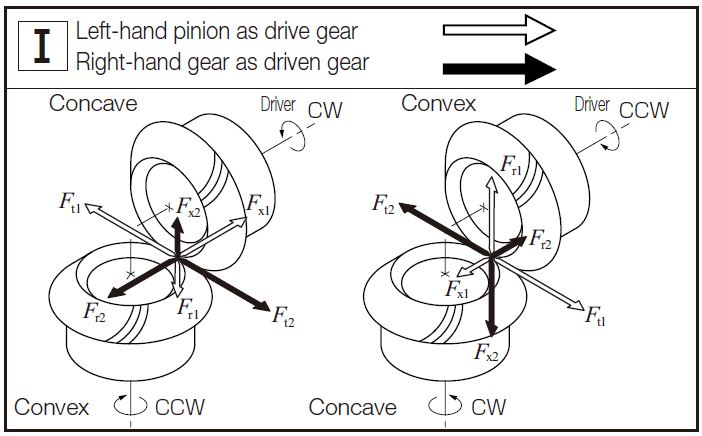
A worm gear box must contain a worm and a mating gear (helical gear) and normally the axis of the worm is perpendicular to the axis of the gear. Look at the picture below:

Where,

Calculations for worm gears are the same as for. Worm Gearing 50 Lead Angle Worm threads are. Perature and the details of the gear mesh design. Worm gear.pdf - Free download as PDF File (.pdf), Text File (.txt) or read. Of a worm gear is related to its circular pitch and number of teeth Z by the formula.

D1 – Pitch Diameter of Worm

D2 – Pitch Diameter of Gear

C – Centre to Centre Distance between the Worm and the Gear

This worm gear design tutorial will discuss up to the selection of the module and pitch and the calculation of the number of teeth, pitch circle diameter and centre to centre distance between the worm and gear. We will use the AGMA formulae for doing the calculations. Design calculations of the other aspects of the worm gear will be discussed in a subsequent part of the tutorial.

Steps of the Design Calculation

The axial pitch of the worm and the circular pitch of the gear must be same for a mating worm and gear. We will use the term Pitch (P) for both the pitch in this tutorial.
Also, the module of the worm as well as the gear must be equal for a mating worm and gear.
Now, let’s say we have the following design input:

Speed of the Worm (N1) = 20 RPM

Speed of the Gear (N2) = 4 RPM

And, we have to find out the Module (m), Pitch (P), Number of helix of Worm (T1), Number of teeth of Gear (T2), Pitch circle diameter of Worm (D1), Pitch circle diameter of Gear (D2), Centre to centre distance(C).

Select the suitable module and its corresponding pitch from the following AGMA specified table:

Module m (in MM) – Pitch P (in MM)

2 ————————-6.238

Worm Gear Calculation

2.5 ———————- 7.854

3.15 ——————— 9.896

4 ————————- 12.566

5 ————————- 15.708

6.3 ———————– 19.792

8 ————————– 25.133

10 ————————- 31.416

12.5 ———————– 39.27

16 ————————– 50.625

20 ————————– 62.832

Say, we are going ahead with the Module as 2 and the Pitch as 6.238.

Use the following gear design equation:

N1/N2 = T2/T1

And, we will get:

T2 = 5 * T1……………….Eqn.1

Now use the following AGMA empirical formula:

T1 + T2 > 40………………Eqn.2

By using the two equations (Eqn.1 & Eqn.2), we will get the approximate values of

T1 = 7 andT2 = 35

Calculate the pitch circle diameter of the worm (D1) by using the below AGMA empirical formula:

D1 = 2.4 P + 1.1

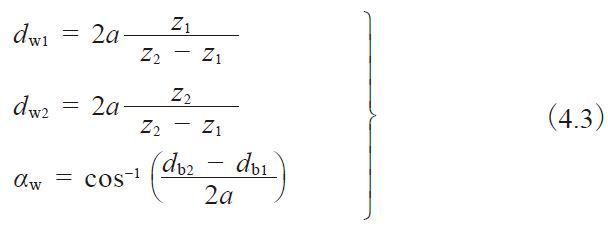
= 16.0712 mm

The following AGMA empirical formula to be used for calculating the pitch circle diameter of the gear (D2):

D2 = T2*P/3.14

= 69.53185 mm

Worm Gear Calculation Formula

Now, we can calculate the centre to centre distance (C) by the following equation:

C = (D1 + D2)/2

= 42.80152 mm

The below empirical formula is the cross check for the correctness of the whole design calculation:

(C^0.875)/2 <= D1 <= (C^0.875)/1.07

Observe that our D1 value is falling in the range.

Conclusion

The worm gear box design calculation explained here uses the AGMA empirical formulas. A few worm gear design calculator are available on web, and some of them are free as well.

In the next worm gear box design calculation tutorial we will discuss the force analysis of a worm gear box.

Worm Gear Design Calculation Pdf Free

Axial tooth pitch

p_x = π_x

Basic tooth pitch

Lead

p_z = z₁ p_x

Virtual/alternate number of teeth

Helix angle at basic cylinder

sin γ_b = sin γ cos α_n

Worm pitch cylinder diameter

Worm gear pitch circle diameter

d₂ = z₂ m_x

Worm outside cylinder diameter

Worm gear outside circle diameter

d_a2 = d₂ + 2m (h_a^* + x)

Worm root cylinder diameter

Worm gear root circle diameter

d_f2 = d₂ - 2m (h_a^* + c^* - x)

Worm rolling(work) circle diameter

Worm gear rolling(work) circle diameter

d_w2 = d₂

Worm gear root circle diameter

Center distance

Chamfer angle of worm gear rim

Worm tooth thickness in normal plane

Worm gear tooth thickness in normal plane

Worm tooth thickness in axis plane

s_x1 = s₁ / cos γ

Worm gear tooth thickness in axis plane

Work face width

b_w = min (b₁, b₂)

Contact ratio

ε_γ = ε_α + ε_β

where:

Minimum worm gear tooth correction

where:

h_a^*₀ = h_a^* + c^* - r_f^* (1 - sin α)

c = 0.3	for α = 20 degrees
c = 0.2	for α = 15 degrees

m_n = m
Normal module	m_x = m_n cos γ
Axial pressure angle	α_x = a
Normal pressure angle	α_n = arctg (tg α cos γ)
Helix/lead angle	γ = arcsin z₁/q

m_n = m_x / cos γ
Normal module	m_x = m
Axial pressure angle	α_n = arctg (tg α cos γ)
Normal pressure angle	α_x = α
Helix/lead angle	γ = arctan z₁/q The configuration details for this step can be found in the installation guide.

Docshunter546